Section Modulus J

Tuesday, September 28, 2021

The polar section modulus also called section modulus of torsion Z p for circular sections may be found by dividing the polar moment of inertia J by the distance c from the center of gravity to the most remote. The moment carrying capacity of an object is directly dependent on geometrical property I and material property E of an objectwhich is collectively termed as flexural rigidity EIGeometry of an object plays an important role in load bearing capacity of an object which is indicated by moment of inertia of a section.

Tapered Tee Beam Geometric Properties

Section Properties Tee Profile Case 33 Calculator.

Section modulus j. Where I moment of inertia y distance from centroid to top or bottom edge of the rectangle. Butt Weld Section Modulus Equation and Calculation Fillet Weld Polar Moment of Inertia Equations and Calculation Fillet Weld Moment of Inertia Equations and Calculation Fillet Weld Throat Area Equations and Calculation Active Fillet Weld Height Specification Weld. For symmetrical sections the value of Z is the same above or below the centroid.

The stress in the outermost section of beam is computed with the help of section modulus. C Distance to Centroid in or mm. Moment of Inertia Section Modulus Radii of Gyration Equations T Sections.

Elastic modulus of upper flange about Y-Y axis. 3222 Effective Section Modulus Having evaluated the compactness of an open section the effective section modulus Z e is then determined. A beam that has a larger section modulus than another will be stronger and capable of supporting greater loads.

Its a property of a shape virtually any shape. Section Modulus Radii of Gyration Equations W and S Profiles. I Second moment of area in 4 or mm 4.

Diameter of a solid shaft can calculated by the formula. J Torsional Constant in 4 or mm 4. Z e is calculated using Clauses 523 524 and 525 of AS 4100.

Surface area per unit weight. D shaft inside diameter m ft Diameter of a Solid Shaft. Calculating the section modulus.

Look at its units cubic inches. The section modulus is a number. 3 d b t3 J 14 3 3 3 36 d b t C w Bleich 1952 Picard and Beaulieu 1991 15 2 2 t b b t d d 16 The warping constant of angles is small and often neglected.

J π D 4 - d 4 32 3b where. Using the section modulus the bending stress is calculated as σ b M S. To calculate the section modulus the following formula applies.

Sy upper flange cm 3. Z max and Z min. It is a direct measure of the strength of the beam.

3 d b t3 J 14 3 3 3 36 d b t C w Bleich 1952 Picard and Beaulieu 1991 15 2 2 t b b t dd 16 The warping constant of angles is small and often neglected. The section modulus of the cross-sectional shape is of significant importance in designing beams. K Radius of Gyration in or mm.

Z Elastic Section Modulus. Calculation of the effective section modulus at yield is described in Chapter 4 of this book. A Geometric Area in 2 or mm 2.

J i Polar Moment of Inertia in 4 or mm 4. That makes no sense right. Thickness of flange or Wall thickness.

C Distance to Centroid in or mm. For asymmetrical sections two values are found. J Polar moment of inertiaCircular Sections m 4 J Polar moment of inertiaNon circluar sections m 4 K Factor replacing J for non-circular sections m 4 r radial distance of point from center of section m r o radius of section OD m τ shear stress Nm 2 G Modulus of rigidity Nm 2 θ angle of twist radians Formulas.

Z Elastic Section Modulus. Section Properties Tee Profile Case 32 Calculator. Torsional section properties fillets neglected.

Hull girder section modulus to the deck often determines the bending strength of. Korashy ii Sy cm 3. Tmax and and for db ratios in excess of 10 kl k.

It has nothing to do with timber stainless steel aluminum plastic or your standard structural steel. P Perimeter of shape in or mm. I Second moment of area in 4 or mm 4.

It includes the idea that most of the work in bending is being done by the extreme fibres of the beam ie the top and bottom fibres of the section. Thickness of gusset plate. It is indicated by S.

J π R 4 2 π D 2 4 2 π D 4 32 3 where. Ck2db3 T kldb2 ie. For double angles the values of J and C w can be taken equal to twice the value.

Technical Notes 3B - Brick Masonry Section Properties May 1993 Abstract. P Perimeter of shape in or mm. This Technical Notes is a design aid for the Building Code Requirements for Masonry Structures ACI 530ASCE 5TMS 402-92 and Specifications for Masonry Structures ACI 5301ASCE 6TMS 602-92Section properties of brick masonry units steel reinforcement and brick masonry assemblages are given to simplify the.

A Geometric Area in 2 or mm 2. K Radius of Gyration in or mm. The utility of the section modulus is that it characterizes the bending resistance of a cross section in a single term.

Ckldb2 and J is the effective polar moment of area or equivalent J see 57 J web J flanges k2dl b. This parameter is based on the section moduli S Z and is used in the determination of the design section moment capacity φM S. D shaft outside diameter m in Polar Moment of Inertia of a circular hollow shaft can be expressed as.

Torsional section properties fillets neglected. For double angles the values of J and C. M s Z ef y 51 where Z e is the effective section modulus about a given axis computed at the yield stress f y.

J Torsional Constant in 4 or mm 4. Surface area per unit length. J i Polar Moment of Inertia in 4 or mm 4.

L k2db3G JG _- where Z is the torsion section modulus Z web Z flanges kldlbt kld2b. The design for a determines the nominal section moment capacity M s given by Eq. Also it is the measure of strength of given member.

Elastic modulus of section about Y-Y axis. It is termed as the ratio of second moment of area and distance from NA Neutral axis to the extreme fiber. Hull girder section modulus is a well-accepted parameter measuring the longitudinal bending strength of ships.

Moment of Inertia Section Modulus Radii of Gyration Equations T Sections. This allows for optimization of a beams cross section to resist bending by maximizing a. The polar moment of inertia may be found by taking the sum of the moments of inertia about two perpendicular axes lying in the plane of the cross-section and passing through this point.

Distance between outer fibers of an angle to V-V axis. This modulus is perhaps the single most important design parameter describing hull girder strength.

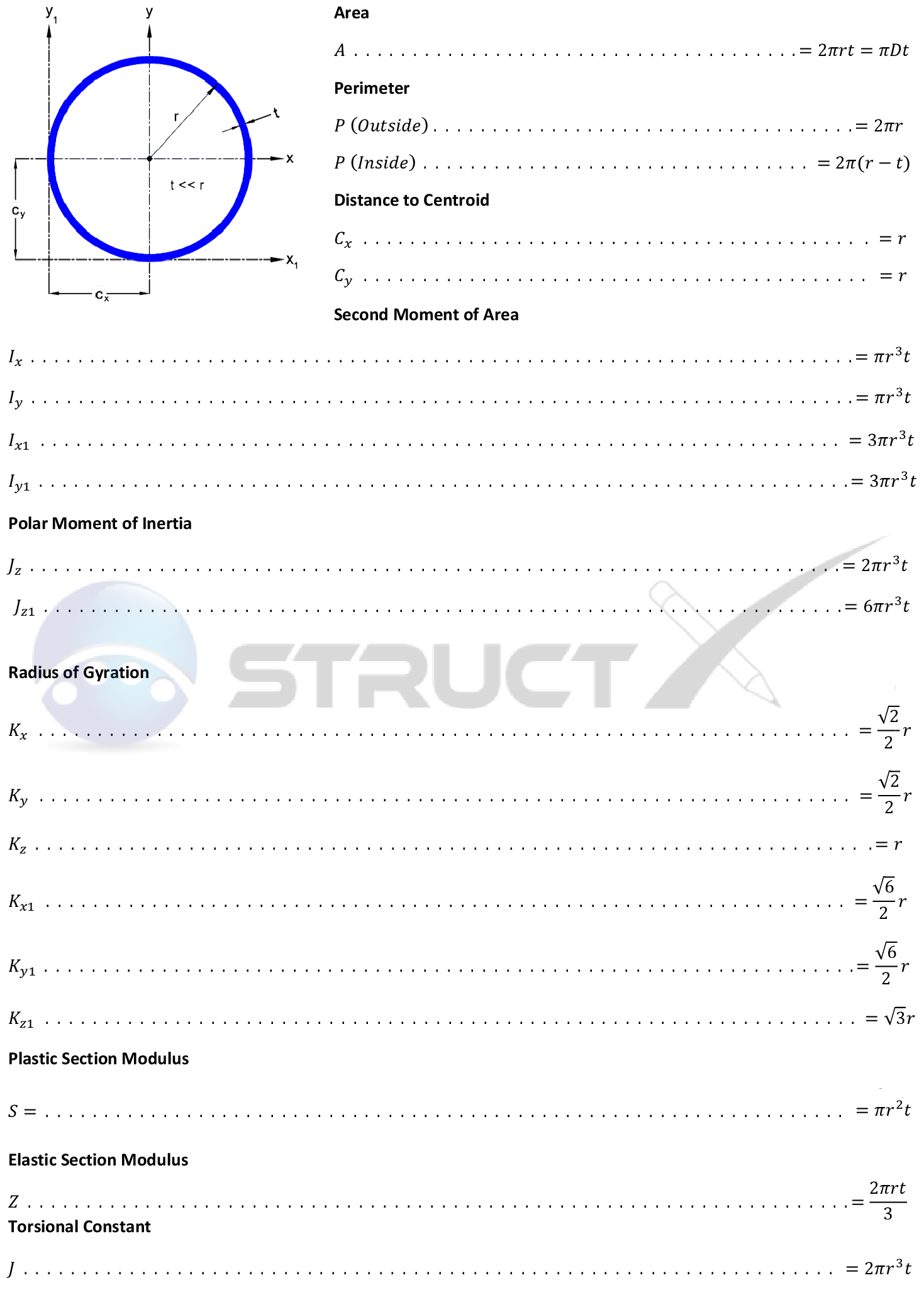
Thin Walled Circle Geometric Properties